In particle physics, we almost always use approximations.

Often, we assume the forces we consider are weak. We use a “coupling constant”, some number written or or , and we assume it’s small, so is greater than is greater than . With this assumption, we can start drawing Feynman diagrams, and each “loop” we add to the diagram gives us a higher power of .

If isn’t small, then the trick stops working, the diagrams stop making sense, and we have to do something else.

Except for some times, when everything keeps working fine. This week, along with Simon Caron-Huot, Lance Dixon, Andrew McLeod, and Georgios Papathanasiou, I published what turned out to be a pretty cute example.

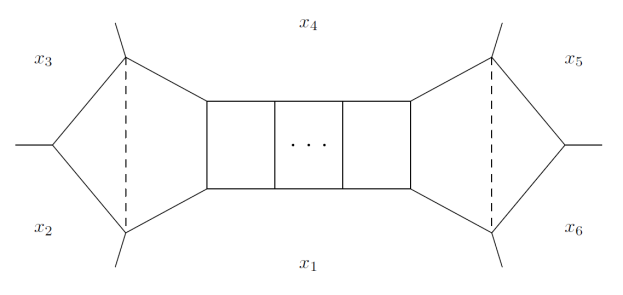
We call this fellow . It’s a family of diagrams that we can write down for any number of loops: to get more loops, just extend the “…”, adding more boxes in the middle. Count the number of lines sticking out, and you get six: these are “hexagon functions”, the type of function I’ve used to calculate six-particle scattering in N=4 super Yang-Mills.

The fun thing about is that we don’t have to think about it this way, one loop at a time. We can add up all the loops, times one loop plus times two loops plus times three loops, all the way up to infinity. And we’ve managed to figure out what those loops sum to.

The result ends up beautifully simple. This formula isn’t just true for small coupling constants, it’s true for any number you care to plug in, making the forces as strong as you’d like.

We can do this with because we have equations relating different loops together. Solving those equations with a few educated guesses, we can figure out the full sum. We can also go back, and use those equations to take the s at each loop apart, finding a basis of functions needed to describe them.

That basis is the real reward here. It’s not the full basis of “hexagon functions”: if you wanted to do a full six-particle calculation, you’d need more functions than the ones is made of. What it is, though, is a basis we can describe completely, stating exactly what it’s made of for any number of loops.

We can’t do that with the hexagon functions, at least not yet: we have to build them loop by loop, one at a time before we can find the next ones. The hope, though, is that we won’t have to do this much longer. The basis covers some of the functions we need. Our hope is that other nice families of diagrams can cover the rest. If we can identify more functions like , things that we can sum to any number of loops, then perhaps we won’t have to think loop by loop anymore. If we know the right building blocks, we might be able to guess the whole amplitude, to find a formula that works for any you’d like.

That would be a big deal. N=4 super Yang-Mills isn’t the real world, but it’s complicated in some of the same ways. If we can calculate there without approximations, it should at least give us an idea of what part of the real-world answer can look like. And for a field that almost always uses approximations, that’s some pretty substantial progress.